-
Equation for a plane spiral:
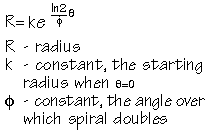
-
Parametric version:
-
Length of Spiral over phi:
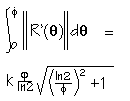
-
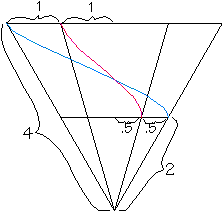
Here are the spirals or helixes over an interval of 180 degrees
These spirals wrap around cones which can be uncurled to wedges.
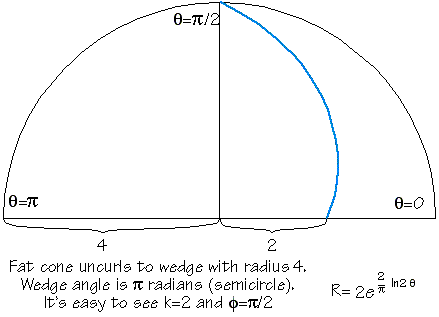
Using equation at top of the page we can get this curve's length.
Let's call it H
H approximately = 4.954
-
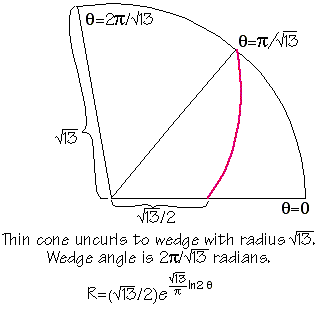
Using equation at top of the page we can get this curve's length.
Let's call it h
h approximately = 2.896
-
Ramp
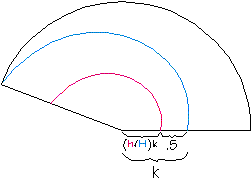
Connecting the fat and thin cones is a surface I call the ramp.
If the ramp could be made from a plane, its boundaries would be parallel spirals,
that is, they have the same center and rate of growth.
-
The inner boundary has the same length as the thin cone spiral (h)
The outer boundary has the same length as the fat cone spiral (H)
-
The starting distance between inner and outer boundaries is .5,
(see illustration at the top of this page)
Therefore starting radius k=.5/(1-h/H)
k=approx 1.203
-
Knowing the starting length k and the length of the spiral,
we can find the angle over which this spiral doubles
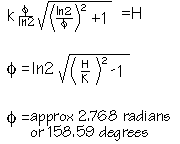
-
I have little doubt that the wall spirals can be made from cone wedges.
I'm not sure the ramp can be made from a flat sheet of paper though.
I am assuming the distance between two points on the flat surface will
equal
the length of the geodesic between the transformed points.
I am not sure this is true but the pieces seem to work in the cardboard
model.